Welcome to the final Quantitative Reasoning article!
Today, I will be going over examples of geometry and trigonometry problems similar to those found on the OAT. As always, I have created each of these free practice problems myself. Our next OAT article series will be covering Physics, as we have received numerous requests for that section.
Note: * always denotes multiplication, and the hat symbol represents powers, i.e. x squared is written as x^2
Problem 1:
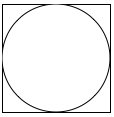
There is a circle inscribed within a square, and the length of one side of the square is equal to 5x. What is the approximate area of the region within the square but outside of the circle? Use 3.14 for the value of π
When we have a circle inscribed within a square, it means that we have a circle with a diameter equal to the length of one side of the square, and the centers of both the circle and the square are at the same point. Here’s what it looks like:
So to solve this problem, we need to find out the area of the circle, the area of the square (ignoring the circle), and then to find the area of the region within the square but outside the circle, we’ll simply subtract the area of the circle from the area of the square. Simple enough, right? We’ll start by finding the area of the square, and the formula for area of a square is simply side times side, which means we’ll have 5x * 5x = 25x^2 (read as twenty five x squared). Now, we need to find the area of the circle. The formula for the area is π times the radius squared. The radius is half the diameter, and we can see that the diameter of the circle is going to be the same length as one side of the square, which is 5x in this case. So half of 5x = 2.5x = the radius of the circle. The square of the radius is 2.5x * 2.5x = 6.25 x^2 (read as 6.25 x squared). The area of the circle then, is going to be 6.25 * π * x^2 and finally, the area outside the circle but within the square is just 25x^2 minus 6.25 * π * x^2
To get the approximate answer using 3.14 as π we will probably just use a calculator, as these are now allowed on the OAT Quantitative Reasoning section. 6.25 * 3.14 = 19.625 so we will end up with 25x^2 minus 19.625x^2 = 5.375x^2 as our final answer.
Problem 2:
A girl is flying a kite. With the girl holding the kite string from ground level, the kite is flying at an altitude of 40 feet, and the distance between the girl and the kite is 50 feet. The girl’s father (also at ground level) is standing directly underneath the kite. How far away is the girl from her father?
The easiest way to solve this problem (if you don’t immediately see the answer) is to draw a simple diagram. We will have three points: the girl, the kite, and her father. And we’re going to draw lines connecting each of the three points to each other, so it should be obvious we’ll be forming a triangle. So first draw a point to represent the girl. Then, we’ll draw another point to represent the father. This second point should be connected by a horizontal line to the first point (because both the girl and her father are at the same altitude).
This horizontal line represents the unknown distance, so we can label it as having length x for now. Then, we’ll draw the final point directly above the second point, because the kite is flying directly above the father.
This vertical line will represent a distance of 40 feet. Now, we’ll connect the third point (the kite) to the first point (the girl) by drawing a line between them, and this line will represent a distance of 50 feet. You should notice that this forms a right triangle, because a horizontal line intersects with a vertical line at one of the points (the father) so that angle will be 90 degrees.
Since we have a right triangle, we can use the Pythagorean theorem of a^2 + b^2 = c^2 to find the length of the unknown side. The hypotenuse must be the side opposite the right angle, so we know that c needs to be 50 feet. We’ll call side b the unknown side, and side a will be the remaining side of 40 feet. Once we make the substitutions, we can solve the equation, using a calculator if necessary. 50*50 equals 2500 and 40*40 equals 1600 and 2500 minus 1600 equals 900. The square root of 900 is 30, so our unknown side is 30 square feet.
There is, of course, a rather nice shortcut for solving this problem. The moment you realize it is a right triangle with a hypotenuse of 50 feet and another side of 40 feet, you might recognize that this triangle is simply a multiple of the common right triangle ‘triplet’ of 3, 4, 5. So it is possible to immediately see that the remaining distance must be 30 feet, but if you didn’t see that, it still isn’t overly difficult to solve the problem the long way.
Problem 3:
Cos(x) divided by cot(x) is equal to which of the following?
A: Tan(x)
B: Sin(x)
C: Sec(x)
D: Csc(x)
E: Cos(x)
This problem illustrates the importance of trigonometric identities on the OAT. You should know what the sine, cosine, tangent, cotangent, secant, and cosecant functions are and you should also be able to combine and interconvert them, as you will in this problem.
To do this problem, we’ll get everything in terms of sine and cosine, and then cancel appropriately to reduce to our final answer. Cos(x) means cosine of x, and we won’t need to touch that. Cot(x), however, needs to be converted. The cotangent of x is the inverse of the tangent of x, and tan(x) is the same as sin(x) divided by cos(x) so the cot(x) must be the cos(x) divided by the sin(x). So in the problem, we’ll have the following: cos(x)-,cos(x)-sin(x)..
The cos(x) over cos(x) will cancel out to one, so we’ll end up with one divided by (one over sin(x)) which just gives us sin(x) so our answer will be choice B.
Problem 4:
What is the secant of 300 degrees? Note that this problem could also be asked as what is the secant of 5π/3 using radians. Also, you can add or subtract 360 degrees (or 2π radians) as many times as you want without affecting the answer, so you should know how to cope with these various possibilities on test day. However, if this gives you too much difficulty, I wouldn’t advise you to go overboard trying to learn this at the expense of other, more important material (of which there is plenty), because there shouldn’t be many problems that involve this. The trigonometric identities, an example of which is given back in problem 3, are much easier and more important to learn.
Note: Some calculators are capable of immediately solving this, of course, but I’m working under the assumption that, just as before, you’ll need to solve this by hand. The on-screen calculator function is only just being introduced to the OAT as I am writing this article, so there’s no way for me to know yet exactly what that calculator can and can’t do.
Now, back to the problem. First of all, the secant is just the inverse of the cosine function, so we want to know what the cosine of 300 degrees is. I do know that the cosine is positive in quadrant 4 (quadrant 4 covers 270 degrees to 360 degrees); we know this because of the handy acronym All Students Take Calculus. All functions are positive in quadrant 1, while sine is positive in quadrant 2, tangent is positive in quadrant 3, and cosine is positive in quadrant 4. So if there were any negative answer choices to this problem, we could immediately eliminate them. To proceed further, I’m going to use the ‘symmetry’ method to find what angle in quadrant 1 has an equivalent cosine to 300 degrees; if you cannot follow this method, I’d strongly suggest you consult a book for other methods. So for the ‘symmetry’ method, I know that the cosine function reaches its apex of 1 at 0 degrees, and so on either side of this (i.e. backing up into quadrant 4 or going forward quadrant 1) the cosine function is decreasing in a symmetrical manner. 300 degrees is 60 degrees away from 360 degrees (or 0 degrees) so 300 degrees will have the same cosine as 60 degrees. We should all have the sine, cosine, and tangent memorized for the following values: 0 degrees, 30 degrees, 45 degrees, 60 degrees, and 90 degrees. The cosine of 60 degrees is equal to 1/2 therefore the cosine of 300 degrees is also equal to 1/2. The inverse of 1/2 is 2, so the secant of 300 degrees is 2.
If you have any questions or comments, please leave a reply at the bottom of this page.
Thanks for reading,
Dale Paynter
Salus Optometry 2014′